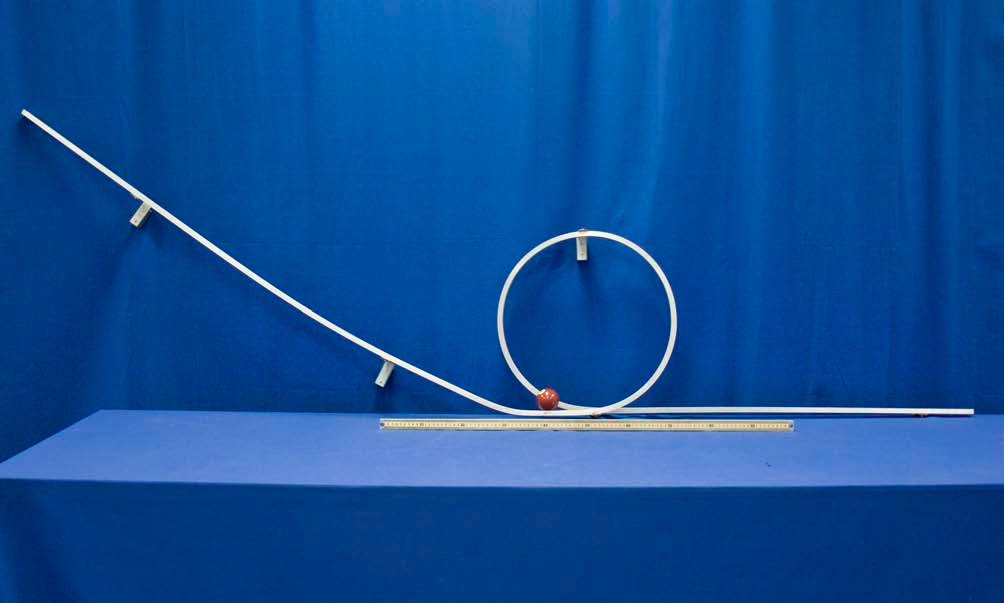


Concept
Assuming the ball is released from rest, what should its minimum initial height be in order to completely orbit the circular loop of radius $R$? This is a classic textbook problem requiring both energy conservation and the analysis of forces at the top of the loop. Neglecting the radius of the ball, $r$, compared to the loop’s radius, $R$, energy conservation from the initial position, $h$, to the loop’s top at $2R$ gives
$$\begin{align} mgh = \frac{1}{2}m v^2 + \frac{1}{2} I \omega^2 + 2mgR \end{align}$$
Now, we know that:
$$\begin{align}I = I_{sphere} = \frac{2}{5} mr^2 \tag{2} \end{align}$$
$$\begin{align}\omega = \frac{v}{r} \tag{3} \end{align}$$
Using equation (2) and (3), we have:
$$\begin{align}gh = \frac{7}{10} v^2 + 2gR \tag{4} \end{align}$$
At the loop’s top, the minimum force required for the ball to just remain in contact with the loop is supplied by gravity without any normal force, so
$$\begin{align} mg = \frac{m v^2}{R} \tag{5} \end{align}$$
$$\begin{align} g = \frac{v^2}{R} \tag{6} \end{align}$$
$(6) \rightarrow (4)$ provides: $h_{min} = 2.7R$ (note that this is $.7R$ above the loop’s top).
Procedure
- Use the magnets on the back of the Loop the Loop Track to anchor it where you want on the whiteboard (as shown in the top-right picture).
- Place the billiard ball on the ramp at the same height as the top of the loop and release it. Notice that it will not make it around the loop because it lacked sufficient kinetic energy to move beyond the loop’s top.
- Now place the billiard ball near the top of the ramp (much higher than the top of the loop) and release it. Notice that it makes it around the loop without falling.
- For a more quantitative demonstration use the meter stick to verify the prediction $h_{min} = 2.7R$.
Equipment
- Loop the Loop Track
- Billiard Ball
- Meter Stick