

Concept
Employing the conservation of mechanical energy between the top and bottom of the incline gives:
$$Mgh = \frac{1}{2}M v^2 + \frac{1}{2}I \left(\frac{v}{R}\right)^2 $$
where $h =$ height, $M =$ mass, $v =$ center of mass velocity, $I =$ moment of inertia about the center of mass, and $R =$ radius. But, I can be expressed as $I = \beta M R^2$, where $\beta$ characterizes the geometry of the given rolling object. Substitution of this expression into the equation for energy conservation gives:
$$v = \sqrt{\frac{2gh}{1+\beta}}$$
where $\beta_{sphere} = \frac{2}{5}$, $\beta_{disc} = \frac{1}{2}$, and $\beta_{ring} = 1$.
Note that the solution is independent of the object’s mass and size, and the winner of a rolling race can be predicted by simply knowing $\beta$.
Procedure
- Choose the geometry of the objects you would like to race down the incline.
- Hold the starting block near the top of the inclined plane so that it blocks the objects from rolling.
- Quickly move the starting block away from you and up to start the objects rolling down the incline.
- Notice that, as long as you are using objects with the same geometry, they reach the bottom of the incline at the same time.
- Repeat as desired using objects with different geometries or uniform densities: small sphere vs. large sphere, small ring vs. large ring, small disc vs. large disc and large aluminum disc vs. wooden disk vs. copper disc.
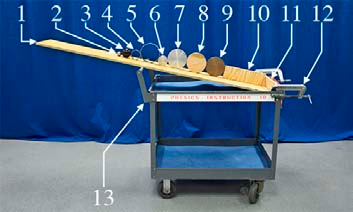
Equipment
- Inclined plane
- Small sphere
- Large sphere
- Small ring
- Large ring
- Small disc
- Large disc
- Wooden disc
- Copper disc
- Starting block
- Finishing block
- (2) C-Clamps
- Cart