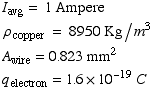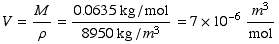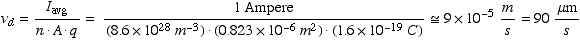CLICK HERE FOR A MORE DETAILED EXPLANATION
1. We can define the AVERAGE CURRENT as the amount of charges  that pass through a point over a time interval
that pass through a point over a time interval  :
:
 (eq. 1)
(eq. 1)
taking the differential limit, we can define the INSTANTANEOUS CURRENT:
 (eq. 2)
(eq. 2)
which is what we usually mean everytime we talk about current.
2. In an electrical circuit, we define charges to be flowing from the POSITIVE END of the battery to the NEGATIVE END, with positive charges as the charge carriers. However, in reality, the charge carriers are actually the ELECTRONS (negative charges), and they "flow" from the negative end to the positive end. The flow direction is defined from + to - for a historical reason: Electric current was observed back in 18th century, but the discovery of electrons was not made until the 19th century. Benjamin Franklin was one of the early scientists who did extensive research on electricity.
3. This brings us to an interesting question: How fast are these charge carriers moving? In other words, what is the drift speed of these electrons?
The calculation of electron drift speed can be found in most college-level undergraduate textbooks (Giancoli, Serway&Jewett, etc), but for the sake of completeness, let's go through the calculation anyway.
First, we start out with our measurable quantity. In an electrical circuit, the easiest to measure is the Current (using an Ammeter), so this is a good place to start our derivation for the drift speed. The average current in an electrical circuit is given by equation 1.
The current we can measure, but the number of charges that pass through, or the time it takes for them to pass through, we cannot measure. Let's try to rewrite these in terms of other variables that might be easier to measure. First, let's take a look at  . If each of the charge carriers carries a charge of q, and we have N charge carriers, that means the total charge
. If each of the charge carriers carries a charge of q, and we have N charge carriers, that means the total charge  is given by
is given by
 (eq. 3)
(eq. 3)
Since the wire is essentially a long cylinder having a certain volume V with N charge carriers in it, we can define the carrier charge density:
 (eq. 4)
(eq. 4)
The charge carriers are moving and therefore will travel a distance  in a period of time
in a period of time  inside the wire. Therefore, the drift speed is given by
inside the wire. Therefore, the drift speed is given by  . If we focus on just this section of the wire (length
. If we focus on just this section of the wire (length  ), then the volume of this section would be
), then the volume of this section would be  . Plugging in this information into the carrier charge density equation (eq. 4), we get:
. Plugging in this information into the carrier charge density equation (eq. 4), we get:
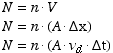
We can plug this back into the expression for equation 3, and then the result into equation 1 to get:
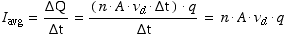
and therefore,
 (eq. 5)
(eq. 5)
We can now use this final expression to calculate the approximate drift speed of the electrons in a wire. Let's take for example, a laptop power cable, which is typically made of an 18-gauge copper wire. This type of cable has a cross-sectional area of  (conversion table). Let's assume that the measured current is 1 Ampere and that each copper atom has 1 free electron. We list the quantities we know:
(conversion table). Let's assume that the measured current is 1 Ampere and that each copper atom has 1 free electron. We list the quantities we know:
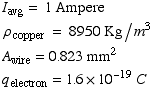
This means we still need fo find the charge carrier density n before we can proceed with our calculation. To find this, we consider the molar mass of the copper, which is 63.5 g/mol. This is the mass of 1 mol of copper. Since we know the density, we can find how much volume 1 mol of copper occupies.
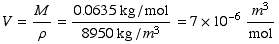
Now, earlier we made the assumption that each copper atom has 1 free electron, which is our charge carrier. Recall that in every mol of a substance, there is an Avogadro's number of atoms. Since we just calculated how much volume 1 mol of copper occupies, that means we have an Avogadro's number of charge carriers in that volume. Using eq. 4, we can obtain:

Plugging this back to eq. 5, we find the approximate drift speed of the electrons:
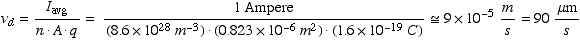
which turns out to be really really small! The charge carriers in this particular wire only moves about a tenth of a millimeter every second, or about a quarter of an inch every minute.
4. If the electron moves that slow, then how come our lightbulbs light up in an instant when we flip the switch? A common misconception is the idea that an electron in a circuit with a bulb actually has to flow from the negative end of the battery all the way to the positive end for the bulb to light up. This is not correct. What actually happens is that the excited electrons move in RANDOM ZIGZAG DIRECTIONS and BUMP into metal atoms inside the wire, transferring the kinetic energy that each electron has and increasing the internal energy of the atoms. When a bulb is connected to the circuit, the electrons increase the internal energy of the atoms in the filament, causing it to heat up and emit light. The velocity that the electrons have while in this random zigzag motion is NOT the drift speed that we calculated earlier. The drift speed is what you get when you average over the velocity of ALL the electrons moving in random directions. The value of the drift speed comes out to be really small because some electrons might be moving towards the positive and some might be moving towards the negative, with a NET MOVEMENT towards the positive. In other words, we have more electrons moving towards the positive than towards the negative, and the NET speed is the drift speed.



 that pass through a point over a time interval
that pass through a point over a time interval  :
: (eq. 1)
(eq. 1) (eq. 2)
(eq. 2) (eq. 3)
(eq. 3) (eq. 4)
(eq. 4) in a period of time
in a period of time  . If we focus on just this section of the wire (length
. If we focus on just this section of the wire (length  . Plugging in this information into the carrier charge density equation (eq. 4), we get:
. Plugging in this information into the carrier charge density equation (eq. 4), we get: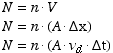
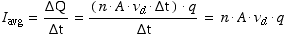
 (eq. 5)
(eq. 5) (
(