Post-Analysis

We verify that the boundary conditions are satisfied. For x > z, $\log{(x/c)} > 0$, and as $t \to t^*$, $d1 \to \infty$ and $d2 \to \infty$. Then both $N(d1) \to 1$ and $N(d2) \to 1$, giving w(x,t^*) = x-c as required. For x < z, $\log{(x/c)} < 0$, and as $t \to t^*$, $d1 \to -\infty$, and $d2 \to -\infty$, so both N(d1) and N(d2)vanish, and w(x,t^*)=0.

To find the number of call options to hold at a given time ( $1/(\partial w/\partial x)$), we calculate

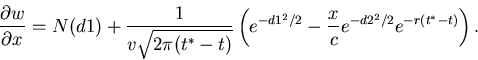$$\frac{\partial w}{\partial x}= N(d1) + \frac{1}{v\sqrt{2\pi(t... ...eft( e^{-d1^2/2}-\frac{x}{c}e^{-d2^2/2} e^{-r(t^*-t)} \right).$$ (34)

If x < c, as $t \to t^*$, $d1 \to \infty$ and $d2 \to \infty$, so $\partial w/\partial x \to 1$, and the number of call options to own at the maturity time t^* is 1. The value of the hedge equity at t^* is then $x - w/(\partial w/\partial x) = x - (x-c)*1 =c$, as it should be.